How To Get Every Network Diagram Question Right On The PMP Exam
This webpage serves as your one-stop Study Guide to help you work through Network Diagram questions wherever you are, even if you don’t have your notes or workbooks nearby.
This Study Guide contains three main parts
- Notes and Definitions
- Statistical Ideas Related to Network Diagrams
- Sample Questions
Within the notes section of this web page, you will find definitions and procedures necessary to solve these questions.
The Statistics section of this page provides a link to my Statistics Study Guide for the PMP Exam. I highly recommend taking a look here when studying, this will be helpful when dealing with more challenging or real-world examples.
Finally, there are a few Sample Questions and solutions where I walk you through solving some of these questions.
Methods Necessary To Solve These Problems
Forward Pass
Let’s assume you have a network diagram. To perform a forward pass we begin at the left. I usually work in columns.
Step 1 : The early start of the first activity is 1. Write 1 on the diagram in the top left corner of Activity C, B and P.
Step 2 : The early finish is equal to the early start plus the duration minus 1. In this example for Activity C the Early finish = 1+6 -1 =5 ; for Activity B the Early finish = 1+4 -1 =4 ; for Activity P the Early finish = 1+3 -1 =3
Step 3. The early start of the next activity (the successor activity) is 1 plus the early finish of the current activity.
For Activity U the early start is 3 + 1 = 4.
Step 4.
Going forward if more than one path converges into a single activity the early start of that activity is 1 plus the largest early finish found on any of the converging paths. When determining the early start of Activity A we will look at the three predecessors. Activity C has a larger early finish than Activity B and P. Therefore to determine the early start of Activity A we will look at the early finish of Activity C only. Early start of Activity A = Early finish of Activity C (6 days) plus 1 day = 7.
(You need to go back to Step 2 to determine the early finish of Activity A and Activity U.)
Step 5. Continue until you have completed the entire forward pass.

Backwords Pass
To perform a backward pass begin at the right and move to the left in columns..
Step 1. You start by using the duration of the critical path (as calculated per above) for the late finishes (LF) of the final activities. In this example In this example, it would include activities T, R, and N.Just copy the early finish of the activity R and write it in the late finish position of activities T, R, and N – the bottom right of these activities T, R and N.
Step 2 : . The late start of an activity is equal to the late finish minus the duration plus 1.
For Activity T the late start =16-2+1=15
For Activity R the late start =16-3+1=14
For Activity N the late start =16-6+1=11
Step 3. The late finish of any immediately preceding activity is the late start of the current activity minus 1. For Activity B the late finish = 15 (which is the late start of Activity E) – 1 = 14
Step 4. The early start of the next activity (the successor activity) is 1 plus the early finish of the current activity.
Going backwards if an activity has two successors we determine its late finish by taking the smallest late start of the successors and subtracting one. For Activity A there are 2 successors (Activity T and R ). Activity R has the smallest late start. The late finish of Activity A = 14 (the late start of Activity R) – 1 = 13.
Step 5. Continue until you have completed the entire backward pass.

Finish to Start (FS) relationships
- This is the most logical and most often used relationship type.
- In this case, A must finish before B starts.
- This is an expression of a relationship between these two activities only.
- For example, the frame of a building must be put up before any electrical work can begin;
- or the rig must be assembled before drilling can begin; or a specification must be written before writing code can begin.

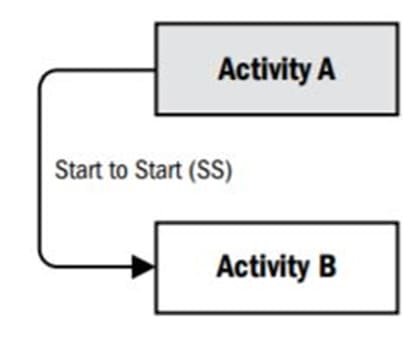
Start to Start (SS) relationships
- This is the relationship when two tasks must begin at the same time.
- For example, as soon work begins on a job site, a nightly watch must be set.
- Another example is when procurement and resource acquisition may both begin at the same time.
- Another way of saying this is: as far as A is concerned, the start of A controls the start of B.
- Many times these are found following a milestone in a project schedule.
Finish to Finish(FF) relationships
- This is when two tasks must finish at the same time.
- One example is two lines of a railroad starting on each coast, with a planned meeting point at some predefined spot and time. In this case, your plan is that both of the lines be completed at the same time. By scheduling this way, you ensure that both crews arrive at the junction point simultaneously, and neither crew has to site idle waiting for the other.
- Another example is obtaining a permit and performing landwork, both of which must be complete prior to drilling. Another way of expressing this is: as far as A is concerned, the finishof A controls the finishof B.
- Many times these are found prior to a milestone in a project schedule.

Start to Finish (SF) relationships
- This is the least common of the relationships, and can be a bit confusing. It means that B may not finish until A starts. A good way to consider this relationship is just in time.
- As an example, imagine that you are building a facility in an area that has no power transmission lines.
- Activity B can be: run a generator to power up site X.
- Activity A can be: turning on a power line to the site, which is being built while the rest of the project progresses. B must continue as long as A has not started. It is not necessary that B finish once A starts, but it is allowed to finish once A starts.
- Another example is when concrete the foundationcannot finish until plumb the foundationstarts—so pipes can come up through the foundation for water lines.

Leads and Lags
- Lead : A lead allows an acceleration of the successor activity. e.g. this could be accomplished by a finish-to-start relationship with a fifteen-day lead Schedule.
For example,
- External Shifting of internet connection takes 4 days from start of order till completion
- You are shifting to your new home in 8 days and you need internet connection in your new home on same day when you shift.
- So you have to lead “apply external shift on day 4 so that you can enjoy the internet on at your new home from day one of shifting
- A lag directs a delay in the successor activity.
- An example would be the time between pouring concrete and sanding it. There would be a 5 day lag on Sanding as a successor to the pouring concrete
- In another example, excavation can begin five days after a permit is submitted. There would be a 5 day lag on Begin Excavation as a successor to the Submit Permit activity

